On Constructing Functions, Part 5
This post is the fifth example in an ongoing list of various sequences of functions which converge to different things in different ways.
Example 1: converges almost everywhere but not in $L^1$
Example 2: converges uniformly but not in $L^1$
Example 3: converges in $L^1$ but not uniformly
Example 4: converges uniformly, but limit function is not integrable
Example 6: converges in $L^1$ but does not converge anywhere

Example 5
A sequence of functions $\{f_n:\mathbb{R}\to\mathbb{R}\}$ which converges to 0 pointwise but does not converge to 0 in $L^1$.
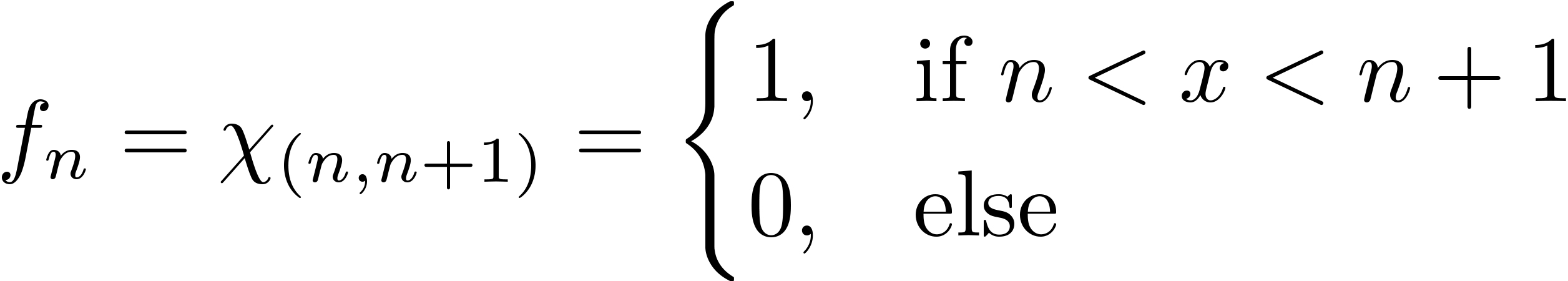
-(resized).gif)
This works because: The sequence tends to 0 pointwise since for a fixed $x\in \mathbb{R}$, you can always find $N\in \mathbb{N}$ so that $f_n(x)=0$ for all $n$ bigger than $N$. (Just choose $N>x$!)
The details: Let $x\in \mathbb{R}$ and fix $\epsilon >0$ and choose $N\in \mathbb{N}$ so that $N>x$. Then whenever $n>N$, we have $|f_n(x)-0|=0<\epsilon$.
Of course, $f_n\not \to 0$ in $L^1$ since $$\int_{\mathbb{R}}|f_n|=\int_{(n,n+1)}f_n=1\cdot\lambda((n,n+1))=1.$$
