Comparing Topologies

It's possible that a set $X$ can be endowed with two or more topologies that are comparable. Over the years, mathematicians have used various  words to describe the comparison: a topology $\tau_1$ is said to be coarser than another topology $\tau_2$, and we write $\tau_1\subseteq\tau_2$, if every open set in $\tau_1$ is also an open set in $\tau_2$. In this scenario, we also say $\tau_2$ is finer than $\tau_1$. But other folks like to replace "coarser" by "smaller" and "finer" by "larger." Still others prefer to use "weaker" and "stronger." But how can we keep track of all of this? Personally, I like to think in terms of (and while sipping a cup of) coffee!

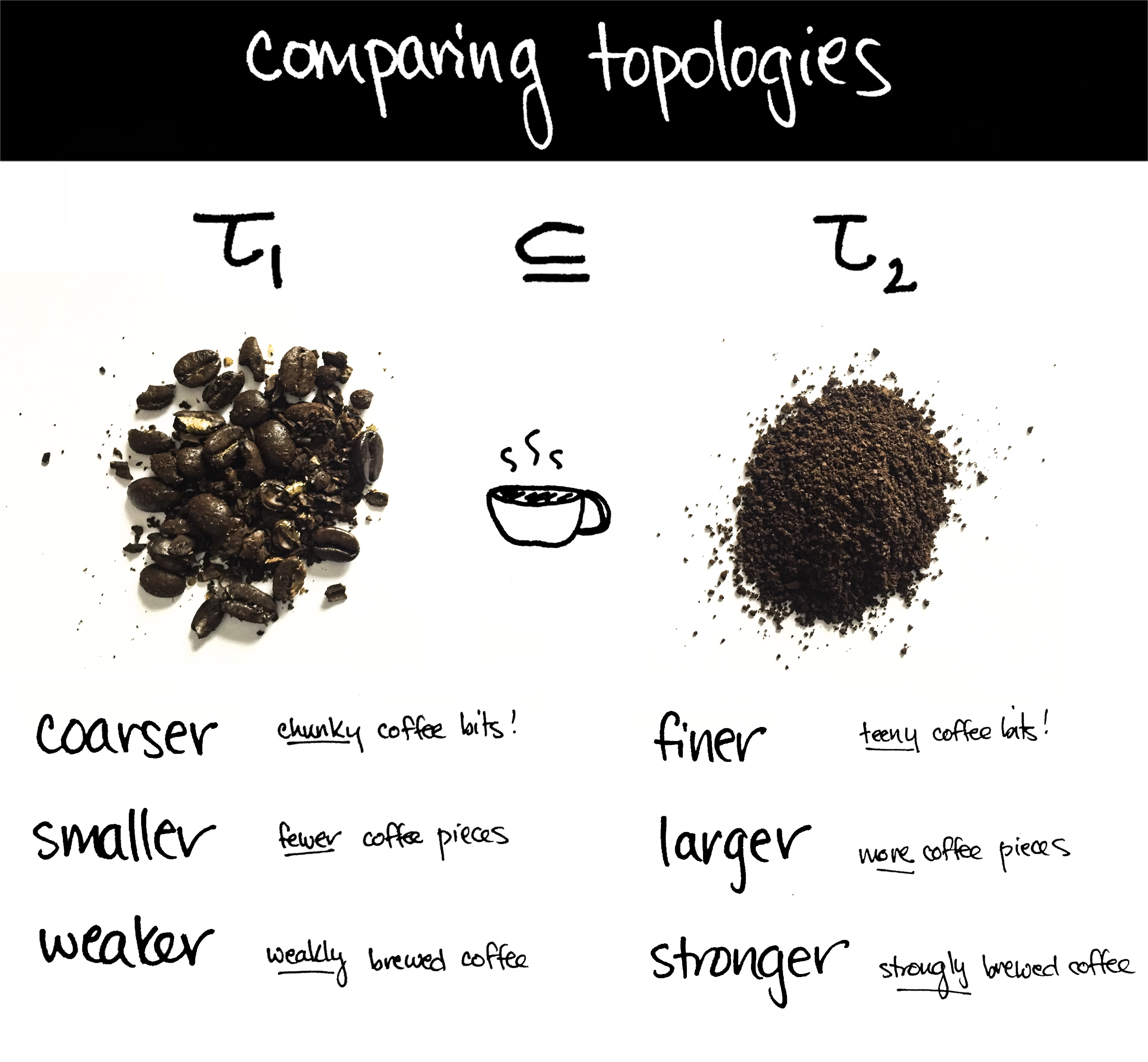
(Now does it make sense why the indiscrete topology on a set $X$ is the coarsest/smallest/weakest topology, while the discrete topology is the finest/largest/strongest topology? )